Someone please lend a hand haha -

Don't see why they're asking to use matrices, it's fairly easy without them (just need a decent diagram).
a) Construct the lunes as follows. Let one of the great circles (call it

) be the "equator", and let the other one
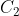
start off the same as the equator, and then "tilt" it by an angle
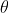
, so half of this second great circle is in the "northern hemisphere" tilted
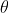
with the equator, and the other half is symmetrically in the "southern hemisphere".
Now, consider the lune in the northern hemisphere with angle
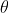
to the equator

(note that the other lune in the northern hemisphere then makes an angle
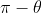
, as the total angle measure of the hemisphere is 180 degrees). The area of this lune is
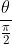
times the area of the lune that goes one quarter of the way around the full sphere (since one quarter of the sphere is 90 degrees, i.e.
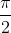
radians). But the area of this "quarter-lune" is just one-quarter the surface area of the sphere, i.e.
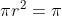
. Therefore, area of the lune of angle
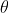
with equator is
=\frac{\theta}{\frac{\pi}{2}} \times \pi = 2\theta)
. Similarly, area of the other lune is
=2(\pi - \theta))
.
Parts b and c not even needed to do part d)
d) Draw a decent diagram of the sphere containing three great circles. You'll see two spherical triangles formed, and these are congruent. Note that each spherical triangle is the intersecting area of three lunes, and there are three pairs of lunes,
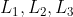
(so two of each

is formed by the three great circles). Let the area of lune

be
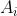
. The angles between adjacent great circles are
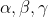
. Therefore,
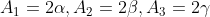
(from part a)). So adding the areas of all lunes present gives
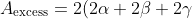)
. This is an "excess" area compared to the surface area of the sphere, because the spherical triangles have been overcounted, as they are intersecting areas of lunes. If we denote the area of one of the spherical triangle by
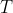
, then
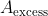
has counted each spherical triangle three times instead of once (so in total, 6T has been added instead of 2T we would want to add to get to the area of the sphere)
Hence in total,
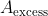
is in excess of the area of the sphere by
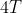
. That is,
 = 4\pi + 4T)
. Solving for
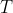
gives

, as required.