Help with a coordinate geometry question. (1 Viewer)

Jonothancroller · Sep 12, 2015

Here is the question:
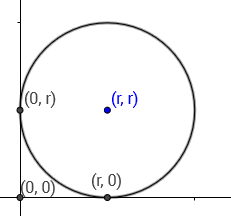
Find the equations of the four circles which are tangent to the x-axis, the y-axis, and the line x + y = 2.
I don't really know where to start. Thanks!

rand_althor · Sep 12, 2015

$Managed to find the equations of the two circles in the first quadrant. Since the x-axis and y-axis are both tangent to the circle, the x-coordinate of the centre is equivalent to the x-intercept of the circle, and the y-coordinate of the centre is equivalent to the y-intercept of the circle. The distance from the centre of the circle to the x-intercept or y-intercept is the radius. This means that the x-intercept is $(r,0)$, the y-intercept is $(0,r)$ and the centre of the circle is $(r,r)$, where $r$ is the radius of the circle.$

$So this means the equation of the circle is $(x-r)^2+(y-r)^2=r^2$. Now the distance from the line $x+y=2$ to the point $(r,r)$ is a radius of the circle. To calculate this distance, we can use the perpendicular distance formula $\left (\frac{\left |Ax_1+By_1+C \right |}{\sqrt{A^2+B^2}} \right )$ as the line from the centre of a circle to a tangent is perpendicular to that tangent. Sub in the line $x+y=2$ and the point $(r,r)$, and let the perpendicular distance be equal to $r$, since this distance is a radius. Solving for $r$, you should get $r=\frac{2}{2-\sqrt{2}},\frac{2}{2+\sqrt{2}}$. Now sub these values into the equation of the circles to get two circles which satisfy the conditions.$

calamebe · Sep 13, 2015

Yeah, and to get the other two you make the centre (-r,r) and (r,-r). Here's my working: m.imgur.com/jKM4MDC sorry for the bad quality.

Edit: I'll just explain why the two other centres are (-r,r) and (r,-r). Though the line x+y-2=0 only passes through the 1st, 2nd and 4th quadrants, then the circle can only be a tangent to the line in those quadrants.

braintic · Sep 13, 2015

Alternatively:

For the two circles in the 1st quadrant, the equation must be of the form:
(x-r)² + (y-r)² = r² since they touch the axes.

Substituting y=2-x and rearranging into a quadratic in x gives:
2x² - 4x + (a-2)² = 0

The discriminant is 16 - 8(a-2)².
Setting this to zero so that there is only one root (ie. one point of intersection with y=2-x) gives the required answer.

Repeat for the other two cases, where the circle has the form:
(x-r)² + (y+r)² = r²
(where r will be negative for the 2nd quadrant circle)

Help with a coordinate geometry question. (1 Viewer)

Jonothancroller

Member

rand_althor

Active Member

calamebe

Active Member

braintic

Well-Known Member

Users Who Are Viewing This Thread (Users: 0, Guests: 1)