seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Here is an interesting problem. If you are not comfortable with higher dimensional spaces, replace "affine hyperplane" with "line" (not necessarily through the origin), and fix n=2 in the below.
Suppose we have finitely many affine hyperplanes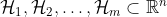
and suppose a point is chosen arbitrarily.
is chosen arbitrarily.
Prove that any sequence defined by:
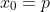
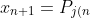}(x_n))
is bounded, where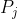 denotes orthogonal projection onto
denotes orthogonal projection onto 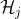 and the sequence
and the sequence ) in
in 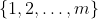 is chosen arbitrarily.
is chosen arbitrarily.
Is your bound independent of your choice of sequence) ?
?
Suppose we have finitely many affine hyperplanes
and suppose a point
Prove that any sequence defined by:
is bounded, where
Is your bound independent of your choice of sequence
Last edited:
