-
Finished your HSC? Check out our University Discussion, Non-School forums or help out next year's HSC students! -
YOU can help the next generation of students in the community! Share your trial papers and notes on our Notes & Resources page
Consecutive roots (1 Viewer)
- Thread starter Aysce
- Start date
ohexploitable
hey
roots are factors of constant term i.e. x=1,2 or x=-1,-2In the quadratic equation, the roots are consecutive. Find the value of m. Thanks !
do same thing for negative roots
Last edited:
Aysce
Well-Known Member
- Joined
- Jun 24, 2011
- Messages
- 2,394
- Gender
- Male
- HSC
- 2012
Cheers, yeah im trying to do 17.b too. Confidence is shattered =.=Since the roots are consecutiveand
are the roots.
When(Consecutive roots)
When(consecutive roots)
Took so long to do.
Im guessing this is the maths in focus textbook cause i just did the question. If you end up doing 17 b) can you tell me how you do it.
Elegantroots are factors of constant term i.e. x=1,2 or x=-1,-2
do same thing for negative roots

Earl Luper
Member
- Joined
- May 18, 2011
- Messages
- 68
- Gender
- Undisclosed
- HSC
- N/A
banging 4 chicks in a row in a night
SpiralFlex
Well-Known Member
- Joined
- Dec 18, 2010
- Messages
- 6,960
- Gender
- Female
- HSC
- N/A
Sure thing.Anyone have questions based on quadratic roots to post? I feel like doing some
M
miserable
Guest
thank u saved my life by solving this Consecutive roots problem ^_^
MrBrightside
Brightest Member
- Joined
- Jan 18, 2010
- Messages
- 2,032
- Gender
- Undisclosed
- HSC
- N/A
is this 3 unit material?
SpiralFlex
Well-Known Member
- Joined
- Dec 18, 2010
- Messages
- 6,960
- Gender
- Female
- HSC
- N/A
For quadratic roots, 2 unit. For cubic and sometimes quartics, 3U.is this 3 unit material?
evanstroeve
Premium Member
- Joined
- Oct 16, 2009
- Messages
- 100
- Gender
- Male
- HSC
- 2011
Nup. 2 unit.is this 3 unit material?
MrBrightside
Brightest Member
- Joined
- Jan 18, 2010
- Messages
- 2,032
- Gender
- Undisclosed
- HSC
- N/A
How did you get the 2nd line? farrr I remember these so faintly.Since the roots are consecutiveand
are the roots.
When(Consecutive roots)
When(consecutive roots)
Took so long to do.
Im guessing this is the maths in focus textbook cause i just did the question. If you end up doing 17 b) can you tell me how you do it.
MrBrightside
Brightest Member
- Joined
- Jan 18, 2010
- Messages
- 2,032
- Gender
- Undisclosed
- HSC
- N/A
therefore a^2 + a = 2Which second line?
a(a+1) =?
SpiralFlex
Well-Known Member
- Joined
- Dec 18, 2010
- Messages
- 6,960
- Gender
- Female
- HSC
- N/A
You must know this for your HSC Mr Brightside.

Let us understand where this actually comes from.
If we have quadratic family of and we will let this be equivalent to each other such that, the roots are and
and 
(x-\beta)=ax^2+bx+c)
Dividing through by
(x-\beta)=x^2+\frac{b}{a}x+\frac{c}{a})
x+\alpha \beta=x^2+\frac{b}{a}x+\frac{c}{a})
By equating coefficients,
=-\frac{b}{a})

That is to say,
The sum of the roots of the quadratic in that form is,
The product of the roots of the quadratic in that form is,
Let us apply this,
For consecutive roots, we can right this as the quadratic having roots,

Now, applying the formula we just created.
The sum of the roots: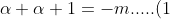)
The produce of the roots are:=2.....(2))
We can now solve for
From)
}{2})
Off it goes into)
}{2}(-\frac{(m+1)}{2}+1)=2)
Solve and find
}{4}=2)


Let us understand where this actually comes from.
If we have quadratic family of and we will let this be equivalent to each other such that, the roots are
Dividing through by
By equating coefficients,
That is to say,
The sum of the roots of the quadratic in that form is,
The product of the roots of the quadratic in that form is,
Let us apply this,
For consecutive roots, we can right this as the quadratic having roots,
Now, applying the formula we just created.
The sum of the roots:
The produce of the roots are:
We can now solve for
From
Off it goes into
Solve and find
Last edited:

