-
Looking for HSC notes and resources? Check out our Notes & Resources page
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
2014 HSC Mathematics Solutions (1 Viewer)
- Thread starter ymcaec
- Start date
Ekman
Well-Known Member
- Joined
- Oct 23, 2014
- Messages
- 1,611
- Gender
- Male
- HSC
- 2015
Hey dude, im a little sceptical about question 15 iii, since everyone at my school (including teachers) are arguing over the proper proof for that question. According to the teachers at my school, you are not allowed to simply use ratios and say A1^2/A^2 = x^2/(x+y)^2 therefore the root of them is equal to what the question wants you to prove. Apparently there are two methods to prove the question. One was either to create an 'imaginary' height of the two triangles, and use these heights of the two triangles and make it equal to the ratio, thus allowing you to sub it in to the area of a triangle formula (0.5 * perpendicular height * base length), giving you the square of the ratio, thus proving the equation, or doing a similar method where instead of using the 'imaginary' height, use the formula of 0.5 * a * b * sin(c) and prove that a and b side lengths of the small triangle are in ratio with the bigger triangle, thus giving you the square of the ratio. I know im being a little vague in my explanation of how the teachers at my school proved this question, but I would like your opinion on this question...
Happy now? No need for any imaginary height.Hey dude, im a little sceptical about question 15 iii, since everyone at my school (including teachers) are arguing over the proper proof for that question. According to the teachers at my school, you are not allowed to simply use ratios and say A1^2/A^2 = x^2/(x+y)^2 therefore the root of them is equal to what the question wants you to prove. Apparently there are two methods to prove the question. One was either to create an 'imaginary' height of the two triangles, and use these heights of the two triangles and make it equal to the ratio, thus allowing you to sub it in to the area of a triangle formula (0.5 * perpendicular height * base length), giving you the square of the ratio, thus proving the equation, or doing a similar method where instead of using the 'imaginary' height, use the formula of 0.5 * a * b * sin(c) and prove that a and b side lengths of the small triangle are in ratio with the bigger triangle, thus giving you the square of the ratio. I know im being a little vague in my explanation of how the teachers at my school proved this question, but I would like your opinion on this question...

Chocolatebubble
Active Member
- Joined
- Sep 13, 2012
- Messages
- 157
- Location
- Sydney, Australia, Australia
- Gender
- Female
- HSC
- 2014
- Uni Grad
- 2019
Omg i did the same thing without Sin A or imaginary height. But I still got the same answers. Would I get marks taken off?Happy now? No need for any imaginary height.

Depends on the criteria, you would prob get zero for part iii) but full marks for part iv) unless you stated why you didn't use sin A or whatever angle used because it is not a right angled triangle.Omg i did the same thing without Sin A or imaginary height. But I still got the same answers. Would I get marks taken off?
I do believe OP's method is valid because it is only worth 2 marks. Seriously, my way was too long for a 2 marker, should be worth 3 or 4
Last edited:
I always think that in maths as long as your method is valid and you get the correct result, they should award you the mark because there is always more than one possible solution.Hey dude, im a little sceptical about question 15 iii, since everyone at my school (including teachers) are arguing over the proper proof for that question. According to the teachers at my school, you are not allowed to simply use ratios and say A1^2/A^2 = x^2/(x+y)^2 therefore the root of them is equal to what the question wants you to prove. Apparently there are two methods to prove the question. One was either to create an 'imaginary' height of the two triangles, and use these heights of the two triangles and make it equal to the ratio, thus allowing you to sub it in to the area of a triangle formula (0.5 * perpendicular height * base length), giving you the square of the ratio, thus proving the equation, or doing a similar method where instead of using the 'imaginary' height, use the formula of 0.5 * a * b * sin(c) and prove that a and b side lengths of the small triangle are in ratio with the bigger triangle, thus giving you the square of the ratio. I know im being a little vague in my explanation of how the teachers at my school proved this question, but I would like your opinion on this question...
Even though writing one line to explain how the result is obtained (as in my solutions) seems like inadequate for 2 marks, but I think the method is valid because that is a property of similar figures (and especially as we have proved similarity in part (i) it is logical to think of this property).
But if one is to use that property, they need to clearly state what the law is because they give you the result to show that you know stuff.
Last edited:
in 13.d.ii isn't the angle supposed to be (360 + 11) - ACB, because point C and point B are not aligned?
The answer might take that into consideration, but its not entirely clear in the answers - any chance for a second opinion?
The answer might take that into consideration, but its not entirely clear in the answers - any chance for a second opinion?
Last edited:
Chocolatebubble
Active Member
- Joined
- Sep 13, 2012
- Messages
- 157
- Location
- Sydney, Australia, Australia
- Gender
- Female
- HSC
- 2014
- Uni Grad
- 2019
not even 1 wittle mark for part iii?Depends on the criteria, you would prob get zero for part iii) but full marks for part iv) unless you stated why you didn't use sin A or whatever angle used because it is not a right angled triangle.
I do believe OP's method is valid because it is only worth 2 marks. Seriously, my way was too long for a 2 marker, should be worth 3 or 4
Yeah I am a bit worried about that as I did exactly what you did in your solutions. I mentioned that the ratio of area is equal to the square of the ratio of sides.I always think that in maths as long as your method is valid and you get the correct result, they should award you the mark because there is always more than one possible solution.
Even though writing one line to explain how the result is obtained (as in my solutions) seems like inadequate for 2 marks, but I think the method is valid because that is a property of similar figures (and especially as we have proved similarity in part (i) it is logical to think of this property).
But if one is to use that property, they need to clearly state what the law is because they give you the result to show that you know stuff.
Hopefully it's valid, but I don't recall being taught that in school so they might not take that as a valid method.
Can someone possibly confirm if you can in fact use that method?.
I remember learning that in year 9 or 10Yeah I am a bit worried about that as I did exactly what you did in your solutions. I mentioned that the ratio of area is equal to the square of the ratio of sides.
Hopefully it's valid, but I don't recall being taught that in school so they might not take that as a valid method.
Can someone possibly confirm if you can in fact use that method?.
in 13.d.ii isn't the angle supposed to be (360 + 11) - ACB, because point C and point B are not aligned?
The answer might take that into consideration, but its not entirely clear in the answers - any chance for a second opinion?
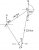

Thus the bearing = 360 - 27.5 = 333 (nearest degree)
which is the same thing as (360 + 11) - ACB
Last edited:
Yay. Hopefully they accept it thenI remember learning that in year 9 or 10
Sports fan 101
New Member
- Joined
- Jan 6, 2014
- Messages
- 12
- Gender
- Male
- HSC
- 2016
A couple of things/stupid errors:
12a) i used Sn formula and simplified to get the sum in terms of n-----would i get 1 mark out of 2???
15a) i got all four solutions but showed no working---would i get 3/3
15c ii) i differentiated y=e^2x and also made y=mx and y=e^2x equal to each other and got x= ln(mx)/2------would i get 1 or 2 out of 3??
16a) i completely fucked this up but i drew a table with x values -pi/3, -pi/6, 0, pi/6, pi/3----would i get 1/3
16ciii) i got x=1.51... and sub this into y to get y=2.3 (approx) would i get e.c.f or have i made question easier----would i get 1 or 2 out of 3
I know its a lot of questions, but would appreciate if anyone could help
12a) i used Sn formula and simplified to get the sum in terms of n-----would i get 1 mark out of 2???
15a) i got all four solutions but showed no working---would i get 3/3
15c ii) i differentiated y=e^2x and also made y=mx and y=e^2x equal to each other and got x= ln(mx)/2------would i get 1 or 2 out of 3??
16a) i completely fucked this up but i drew a table with x values -pi/3, -pi/6, 0, pi/6, pi/3----would i get 1/3
16ciii) i got x=1.51... and sub this into y to get y=2.3 (approx) would i get e.c.f or have i made question easier----would i get 1 or 2 out of 3
I know its a lot of questions, but would appreciate if anyone could help
12a) MaybeA couple of things/stupid errors:
12a) i used Sn formula and simplified to get the sum in terms of n-----would i get 1 mark out of 2???
15a) i got all four solutions but showed no working---would i get 3/3
15c ii) i differentiated y=e^2x and also made y=mx and y=e^2x equal to each other and got x= ln(mx)/2------would i get 1 or 2 out of 3??
16a) i completely fucked this up but i drew a table with x values -pi/3, -pi/6, 0, pi/6, pi/3----would i get 1/3
16ciii) i got x=1.51... and sub this into y to get y=2.3 (approx) would i get e.c.f or have i made question easier----would i get 1 or 2 out of 3
I know its a lot of questions, but would appreciate if anyone could help
15a) No working potentially can lose 2 marks since you showed no process
15c)(ii) You'll get a mark for equating the derivatives but idk if they will accept that form for P so potential loss of 1-2 marks
16a)(i) You get one mark if your table is correct.
16c)(iii) You will get two marks since your process is correct, however the last mark will be determined by the criteria whether they want an exact value or values of 3 sig figs.
Sports fan 101
New Member
- Joined
- Jan 6, 2014
- Messages
- 12
- Gender
- Male
- HSC
- 2016
Thanks heaps.12a) Maybe
15a) No working potentially can lose 2 marks since you showed no process
15c)(ii) You'll get a mark for equating the derivatives but idk if they will accept that form for P so potential loss of 1-2 marks
16a)(i) You get one mark if your table is correct.
16c)(iii) You will get two marks since your process is correct, however the last mark will be determined by the criteria whether they want an exact value or values of 3 sig figs.
Study notes
Member
- Joined
- Jul 2, 2013
- Messages
- 41
- Gender
- Male
- HSC
- 2014
Probably 95 @ person above me
