Complex proofs (1 Viewer)

Run hard@thehsc · Jan 25, 2022

Part II especially

ExtremelyBoredUser · Jan 25, 2022

Run hard@thehsc said:
View attachment 34786
Part II especially

(i)

Triangle OMN is equilateral as indicated by equal sides and therefore the angles within the triangle are all pi/3 or 60 degrees (whichever picks your fancy). OM = ON as sides are equal however OM is the side ON rotated 60 degrees anticlockwise as the angle subtended between OM and ON is 60 degrees. Therefore a rotation 60 degrees anticlockwise of ON will be the same as OM.

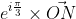
This is mathematically equivalent to $e^{i\frac{\pi}{3}} \times \vec{ON}$ = $\alpha \times n$

(ii)

Triangle OAB is equilateral just like OMN as the sides are equal (as indicated from the diagram). We have to prove that the magnitudes of both vectors are equal (as there is no vector hat on the vectors, this indicates they're only looking for magnitudes but more newer papers have the modulus sign)

I'm going to decompose these vectors into something we can work with;

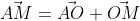
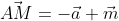
$\vec{AM} = \vec{AO} + \vec{OM}$ , $\vec{BN} = \vec{BO} + \vec{ON}$

$\vec{AM} = -\vec{a} + \vec{m}$ , $\vec{BN} = -\vec{b} + \vec{n}$

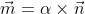
from (i), $\vec{m} = \alpha \times \vec{n}$ and same reasoning to (i), $\vec{a} = \alpha \times \vec{b}$

$\vec{AM} = -\alpha \times \vec{b} + \alpha \times \vec{n}$
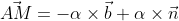
factorising...
$\vec{AM} = \alpha \times (-\vec{b} + \vec{n})$

$\vec{AM} = \alpha \times (\vec{BN})$

Hence,

$| AM | = | BN |$

as required

Complex proofs (1 Viewer)

Run hard@thehsc

Well-Known Member

ExtremelyBoredUser

Bored Uni Student

Users Who Are Viewing This Thread (Users: 0, Guests: 1)