sam davis hostage
New Member
- Joined
- Sep 12, 2023
- Messages
- 2
- Gender
- Male
- HSC
- 2024
I've been looking at some solutions for this question, but they seem all incorrect. Videos include:
In the description of the video by Mind Your Decisions, there is a link to a tiktok by a James Ruse student who also provides a solution. I have a picture of their work attached below.
At some point, they all write something along the lines of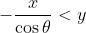 where
where  . However, from this, they incorrectly conclude that
. However, from this, they incorrectly conclude that  , which comes from subbing in
, which comes from subbing in  . This is incorrect, because
. This is incorrect, because 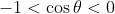 . The correct conclusion should be that
. The correct conclusion should be that 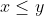 , and the final region should have a solid line diagonal line below the origin. For example, if
, and the final region should have a solid line diagonal line below the origin. For example, if 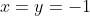 , then
, then 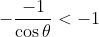 will still be true, as
will still be true, as  . If you go to the desmos and add "/yf1efslc4b" after "calculator" in the url, you can see that the region should include y=x by dragging the z slider around.
. If you go to the desmos and add "/yf1efslc4b" after "calculator" in the url, you can see that the region should include y=x by dragging the z slider around.
I can't tell if this is a misunderstanding on my end or a collective error that everyone made. Any ideas?

At some point, they all write something along the lines of
I can't tell if this is a misunderstanding on my end or a collective error that everyone made. Any ideas?


