part ii)
The question states that we should use the result from part i. Lets use the statement that n is divisible by 4, that is we suppose that
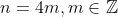
.
Then we put that into the result from part i,
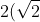^{4m}\cos(m\pi)=2(\sqrt{2})^{4m}(-1)^{m})
convert it back into terms of n,
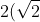^{n}(-1)^{\frac{n}{4}})
.
Now the LHS of that question contains a bunch of n choose expressions, which suggests binomial expansion, so we will expand
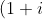^n+(1-i)^n\\\\={n \choose 0}+i{n \choose 1}-{n \choose 2}-i{n \choose 3}+{n \choose 4}+...+{n \choose n}+{n \choose 0}-i{n \choose 1}-{n \choose 2}+i{n \choose 3}+{n \choose 4}+...+{n \choose n})
which simplifies to
)
now equating these two new expressions gives us the answer.