The radical symbol (√) is just square root i.e. to the power of 1/2. In the complex field, √-1, its just i, otherwise i.e. in the real number field, it is impossible to square root negative numbers, hence we write no solution. As for -√1, its -i, much like 1 and -1. In reality, we use i was invented because in the past, imaginary numbers were taboo and people didn't like the thought of square rooting a negative number, pioneers of mathematics and complex numbers decided to make i denote √-1. Nowadays its just to follow convention plus its easier and much neater to write -8i than √-64. In any case, we only really convert from √-1 to i when simplifying the square root of a number like after using the quadratic equation and to put numbers in a+bi form.
 and not
and not  .
.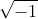 evaluate to?
evaluate to? 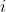 or
or 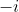 ?
?