more proof (1 Viewer)

Sylfiphy · Feb 23, 2024

prove: sin3x-sinx = 2sinx-4sin³x

here's what I have so far:

LHS = 2cos3x+x/2 sin 3x-x/2
= 2cos2xsinx

RHS= 2sinx-4sin³x
= 2sinxcos2x

so LHS=RHS

but its wrong and idk why🥲

liamkk112 · Feb 23, 2024

Sylfiphy said:
prove: sin3x-sinx = 2sinx-4sin³x

here's what I have so far:

LHS = 2cos3x+x/2 sin 3x-x/2
= 2cos2xsinx

RHS= 2sinx-4sin³x
= 2sinxcos2x

so LHS=RHS

but its wrong and idk why🥲

ideally you should use the sin angle sum formula, that is sin(a+b) = sinacosb+sinbcosa.
then lhs = sin3x-sinx= sin(2x+x)-sinx = sin2xcosx+sinxcos2x-sinx
=2sinxcos^2x+sinx(1-2sin^2x)-sinx from double angle formulas
= 2sinx(1-sin^2x)-2sin^3x from pythagorean identity
=2sinx-4sin^3x=RHS

Average Boreduser · Feb 23, 2024

Sylfiphy said:
prove: sin3x-sinx = 2sinx-4sin³x

here's what I have so far:

LHS = 2cos3x+x/2 sin 3x-x/2
= 2cos2xsinx

RHS= 2sinx-4sin³x
= 2sinxcos2x

so LHS=RHS

but its wrong and idk why🥲

continue on w ur lhs. ur literally like 1 step away dud

Average Boreduser · Feb 23, 2024

liamkk112 said:
ideally you should use the sin angle sum formula, that is sin(a+b) = sinacosb+sinbcosa.
then lhs = sin3x-sinx= sin(2x+x)-sinx = sin2xcosx+sinxcos2x-sinx
=2sinxcos^2x+sinx(1-2sin^2x)-sinx from double angle formulas
= 2sinx(1-sin^2x)-2sin^3x from pythagorean identity
=2sinx-4sin^3x=RHS

spoiler alert

Average Boreduser · Feb 23, 2024

Sylfiphy said:
prove: sin3x-sinx = 2sinx-4sin³x

here's what I have so far:

LHS = 2cos3x+x/2 sin 3x-x/2
= 2cos2xsinx

RHS= 2sinx-4sin³x
= 2sinxcos2x

so LHS=RHS

but its wrong and idk why🥲

lhs= 2cos2xsinx
expand ur cos2x,
lhs= 2(1-2sin^2x)*sinx
continue on from there.

Sylfiphy · Feb 23, 2024

Average Boreduser said:
continue on w ur lhs. ur literally like 1 step away dud

I always realise after someone humbles me but appreciate it yo

Sylfiphy · Feb 23, 2024

liamkk112 said:
ideally you should use the sin angle sum formula, that is sin(a+b) = sinacosb+sinbcosa.
then lhs = sin3x-sinx= sin(2x+x)-sinx = sin2xcosx+sinxcos2x-sinx
=2sinxcos^2x+sinx(1-2sin^2x)-sinx from double angle formulas
= 2sinx(1-sin^2x)-2sin^3x from pythagorean identity
=2sinx-4sin^3x=RHS

thank uuuuuu

Luukas.2 · Feb 23, 2024

This thread actually illustrates how identity problems allow for multiple solutions, some quicker than others:

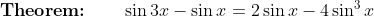
$\textbf{Theorem:} \qquad \sin{3x} - \sin{x} = 2\sin{x} - 4\sin^3{x}$

Proof 1: Conventional LHS / RHS approach

$\begin{align*} \text{LHS} &= \sin{3x} - \sin{x} \\ &= \sin{(2x + x)} - \sin{x} \\ &= \sin{2x}\cos{x} + \cos{2x}\sin{x} - \sin{x} \\ &= 2\sin{x}\cos{x} \times \cos{x} + \left(1 - 2\sin^2{x}\right)\sin{x} - \sin{x} \qquad \text{using the double angle formulae} \\ &= \sin{x}\left(2\cos^2{x} + 1 - 2\sin^2{x} - 1\right) \\ &= \sin{x}\left[2\left(1 - \sin^2{x}\right) - 2\sin^2{x}\right] \qquad \text{using the Pythagorean identity $\sin^2{\theta} + \cos^2{\theta} = 1$} \\ &= \sin{x}\left(2 - 2\sin^2{x} - 2\sin^2{x}\right) \\ &= 2\sin{x} - 4\sin^3{x} \\ &= \text{RHS} \end{align*}$

Proof 2: LHS / RHS approach making use of a sums-to-products formula

$\begin{align*} \text{LHS} &= \sin{3x} - \sin{x} \\ &= 2\sin{\frac{3x - x}{2}}\cos{\frac{3x + x}{2}} \qquad \text{as $\sin{A} - \sin{B} = 2\sin{\frac{A - B}{2}}\cos{\frac{A + B}{2}}$} \\ &= 2\sin{x}\cos{2x} \\ &= 2\sin{x}\left(1 - 2\sin^2{x}\right) \qquad \text{using the double angle formula $\cos{2\theta} = 1 - 2\sin^2{\theta}$} \\ &= 2\sin{x} - 4\sin^3{x} \\ &= \text{RHS} \end{align*}$

Proof 3: Direct Proof without LHS / RHS

$\begin{align*} \sin{3x} = \sin{(2x + x)} &= \sin{2x}\cos{x} + \cos{2x}\sin{x} \\ &= 2\sin{x}\cos^2{x} + \left(\cos^2{x} - \sin^2{x}\right)\sin{x} \qquad \text{using the double angle formulae} \\ &= 3\sin{x}\cos^2{x} - \sin^3{x} \\ &= 3\sin{x}\left(1 - \sin^2{x}\right) - \sin^3{x} \qquad \text{using the Pythagorean identity $\sin^2{\theta} + \cos^2{\theta} = 1$} \\ \text{Thus,} \qquad \sin{3x} &= 3\sin{x} - 4\sin^3{x} \\ \\ \text{Hence,} \qquad \sin{3x} - \sin{x} &= 2\sin{x} - 4\sin^3{x} \qquad \text{as required} \end{align*}$

Drongoski · Feb 24, 2024

more proof (1 Viewer)

Sylfiphy

Member

liamkk112

Well-Known Member

Average Boreduser

Rising Renewal

Average Boreduser

Rising Renewal

Average Boreduser

Rising Renewal

Sylfiphy

Member

Sylfiphy

Member

Luukas.2

Well-Known Member

Drongoski

Well-Known Member

Users Who Are Viewing This Thread (Users: 0, Guests: 1)