In general, we reject the null hypothesis if the p-value is less than the significance level α.
If the alternative hypothesis
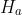
contains the not‐equal‐to symbol (≠), the hypothesis test is a two‐tailed test. Otherwise, all other tests are one-tailed.
If needed, below are solutions to each part, based on the above information. As cossine mentioned, definitely try to attempt the question(s) first to see if you can apply your understanding in your working:
Part (a):
Suppose
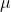
is the average age of Sydney residents; then

is
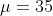
, and
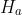
is
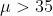
. Note that this is a one-tailed hypothesis test. Here, the p-value 0.0170 is less than the significance level
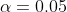
. So we reject the null hypothesis.
Conclusion: this sample information does indicate that the mean age in Sydney has increased from 35 years.
Part (b):
Let
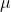
be the average weight of this population; then

is
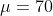
, and
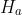
is

. This is a one-tailed test. The standard deviation of weights in this population is
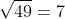
. Here, the p-value 0.001 is less than the significance level
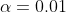
. So we reject the null hypothesis.
Conclusion: the sample data do provide enough evidence for us to conclude that the mean weight for the population is less than 70 kg.
Part (c):
Let
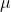
be the average IQ score of this population; then

is
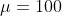
, and
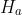
is
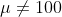
. This is a two-tailed test. Here, the p-value 0.095 is greater than the significance level
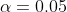
. So we do not reject the null hypothesis.
Conclusion: on the basis of these data, we cannot conclude that the mean IQ score for this population is not 100.
I hope this helps!

contains the not‐equal‐to symbol (≠), the hypothesis test is a two‐tailed test. Otherwise, all other tests are one-tailed.
is the average age of Sydney residents; then
is
, and
is
. Note that this is a one-tailed hypothesis test. Here, the p-value 0.0170 is less than the significance level
. So we reject the null hypothesis.
be the average weight of this population; then
is
, and
is
. This is a one-tailed test. The standard deviation of weights in this population is
. Here, the p-value 0.001 is less than the significance level
. So we reject the null hypothesis.
be the average IQ score of this population; then
is
, and
is
. This is a two-tailed test. Here, the p-value 0.095 is greater than the significance level
. So we do not reject the null hypothesis.


