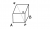totallybord
Member
- Joined
- Mar 13, 2006
- Messages
- 209
- Gender
- Female
- HSC
- 2008
Hi,
Just trying to explain this to cousin but cannot.
The Question is in 2G question 14 part 2 of a. The diagonal PQ of the rectangular prism in the diagram makes angles of alpha, beta and gamma respectively with the edges PA, PB and PC.
(diagram is rectangular prism with base labelled from front left A, P and B and top face Q in the back left corner and C in the front right corner. No other letters are given)
Prove that cos^2alpha+cos^2beta+cos^2gamma=1.
i)This has been proven.
ii) What is the 2 dimensional version of this result? This is the question that I didn't understand. It says in the answers that cos^2alpha+cos^2beta=1 where alpha +beta=90degrees
Please explain.
Thanks!
Just trying to explain this to cousin but cannot.
The Question is in 2G question 14 part 2 of a. The diagonal PQ of the rectangular prism in the diagram makes angles of alpha, beta and gamma respectively with the edges PA, PB and PC.
(diagram is rectangular prism with base labelled from front left A, P and B and top face Q in the back left corner and C in the front right corner. No other letters are given)
Prove that cos^2alpha+cos^2beta+cos^2gamma=1.
i)This has been proven.
ii) What is the 2 dimensional version of this result? This is the question that I didn't understand. It says in the answers that cos^2alpha+cos^2beta=1 where alpha +beta=90degrees
Please explain.
Thanks!