HSC 2012 MX2 Marathon (archive) (1 Viewer)

SpiralFlex · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

First one is straight forward from textbook and HSC paper.

Second one. After substitution plus partialness. $=\frac{1}{\sqrt{2}}\tan^{-1}(\tan x - \cot x)+\frac{1}{2\sqrt{2}}\ln\frac{\tan x - \cot x-x}{\tan x - \cot x+x}$

^This? I am leaving the forum if I don't get this answer correct. I've been puzzled by this for a while now. I hope I am not over thinking.

Must be an easier way haha AAEldar.

Note: Sir AAEldar gave me this question a while back.

Carrotsticks · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

SpiralFlex said:
^This? I am leaving the forum if I don't get this answer correct. I've been puzzled by this for a while now. I hope I am not over thinking.

You forgot the +C.

Now get out of the forums.

SpiralFlex · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Carrotsticks said:
You forgot the +C.

Now get out of the forums.

asdogfajogjagrddgs

mnmaa · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

how do you guys "write mathematical notation and symbols on here. I'm currently stuck to using z^2

SpiralFlex · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

LATTTEEEEXXXXXXX

To start of it's best to use an equation editor until you become fluent.

http://www.codecogs.com/latex/eqneditor.php

Simply add [tex.] to the beginning. And [/tex.] to the end. (no dots.)

Carrotsticks · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

mnmaa said:
how do you guys "write mathematical notation and symbols on here. I'm currently stuck to using z^2

http://community.boredofstudies.org/showthread.php?t=234259

mnmaa · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Carrotsticks said:
http://community.boredofstudies.org/showthread.php?t=234259

Thanks brah

SpiralFlex · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Wait I made a mistake in one of my lines.

I will fix and re-try later.

Carrotsticks · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

I got two methods of doing it. I'm feeling quite tired right now, so I'll just outline the general idea. I might complete it some time later.
Method #1:

$u^{2}= \tan(x)$

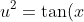... that the integral simplifies to

$\int \frac{u^{2}}{1+u^{4}}$

Then proceed to manipulate (which will involve dividing all terms by u^2 at some point), then use another substitution

$k=u+\frac{1}{u}$

And also:

$k=u-\frac{1}{u}$

Which will lead to the inverse tan integrals, in terms of K.

Method #2: (this one is a bit longer)

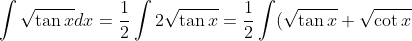$\int \sqrt{\tan x} dx = \frac{1}{2} \int 2 \sqrt{\tan x} = \frac{1}{2} \int (\sqrt{\tan x} + \sqrt{\cot x}) + (\sqrt{\tan x} - \sqrt{\cot x})$

Split as two integrals, then turn all terms into sin and cos.

After a whole lot of manipulation, you will come across a situation where you will need the substitutions:

$u=\sin x - \cos x$

And similarly:

$v=\sin x + \cos x$

Then we will get a log standard integral and an inverse sine integral.

Oh and don't forget the +C (Spiral).

mnmaa · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Ive discovered zoho documents, its an app on google chrome and is much more user friendly and easier to learn the latex

AAEldar · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Carrotsticks said:
Method #1:

$u^{2}= \tan(x)$

... that the integral simplifies to

$\int \frac{u^{2}}{1+u^{4}}$

Then proceed to manipulate (which will involve dividing all terms by u^2 at some point), then use another substitution

$k=u+\frac{1}{u}$

And also:

$k=u-\frac{1}{u}$

Which will lead to the inverse tan integrals, in terms of K.

That's the way I did it. I had another way very similar to that as well but it was too messy so I gave up hahaha.

Hadn't thought of your method 2 though!

mnmaa · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

factorise Z⁵-1=0, Into real linear and quadratic roots.

Carrotsticks · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

We've had very few curve sketching ones.

Consider the following graph y=f(x). The asymptote occurs at x=-1.5

Draw the graph of:

$$a) $ y=f \left (\frac{1}{x} \right )$

$$b) $ y=f(x^{2})$

$$c) $ y=f \left (e^{f(x)} \right)$

cutemouse · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

Carrotsticks said:
I've seen this question before... I don't quite remember when.

It's a common question. But there is a very elegant solution to it, ie. without using arguments.

Carrotsticks · Feb 7, 2012

Re: 2012 HSC MX2 Marathon

cutemouse said:
It's a common question. But there is a very elegant solution to it, ie. without using arguments.

I will attempt to find said solution. I don't like the T. Lee solutions.

I attempted two solutions earlier today.

First one involved constructing an arbitrary chord within the circle that goes through all 3 points. However, I made sure that all 3 points were on the same half of the circle that the chord divides. I then utilised the property that angles subtended from the same arc are equal. However, the algebra got a bit messy.

Second one involved defining some point P as the centre of the circle. I then used the fact that Pz_1, Pz_2 and Pz_3 were equal to try to establish an identity that would lead to showing that they are collinear but again, the algebra got messy.

math man · Feb 8, 2012

Re: 2012 HSC MX2 Marathon

Yeh I have proved that question numerous ways,
Including the good old fashion gradient AB = gradient
BC way, whIch doesn't involve arguments, but I Feel
If you understand thE argument method then you should
Use it, besides the question said they were concyclic points
MeanIng the question was intended to use circle geo therefore
Using argumentS

Carrotsticks · Feb 8, 2012

Re: 2012 HSC MX2 Marathon

Consider the Ellipse in canonical form. Around it is the auxiliary circle (radius a)

From an arbitrary point on this circle, a chord of contact to the ellipse is drawn.

Find the locus of the midpoint of this chord.

Carrotsticks · Feb 8, 2012

Re: 2012 HSC MX2 Marathon

cutemouse, I found two alternative proofs for the question you posted.

Proof #1

$\\ $Consider 3 arbitrary points in the Complex Plane $ z_1,z_2,z_3 \\\\ $Define 3 vectors: $ \\\\ Z_{i<j} = \frac{1}{z_i} - \frac{1}{z_j}, i=1, j=3 \\\\ $Apply the Triangle Inequality: $ \\\\ \left | \frac{1}{z_1} - \frac{1}{z_3} \right | \leq \left | \frac{1}{z_1} - \frac{1}{z_2} \right | + \left | \frac{1}{z_2} - \frac{1}{z_3} \right | \\\\ $Combine the fractions:$ \\\\ \frac{\left | z_1 - z_3 \right |}{\left | z_1 \right | \left | z_3 \right |} \leq \frac{ \left | z_1 - z_2 \right |}{\left |z_1 \right | \left | z_2 \right |} + \frac{\left | z_2 - z_3 \right |}{\left | z_2 \right | \left | z_3 \right |} \\\\ $Multiply both sides by $ \left | z_1 \right | \left | z_2 \right | \left | z_3 \right |: \\\\ \left | z_2 \right | \left | z_1 - z_3 | \right | \leq \left | z_3 \right | \left | z_1 - z_2 \right | + \left |z_1 \right | \left |z_2 - z_3 \right |$

We recognise Ptolemy's Inequality, where equality occurs when the 3 points are concyclic.

However, equality also occurs when the points 1/z_k, k=1,2,3 lie on the same line.

Hence if the 3 points are concyclic, their reciprocals are collinear.

Proof #2

We recognise that the mapping...

$z \rightarrow \frac{1}{z}$

... is a Mobius Transform (http://en.wikipedia.org/wiki/Möbius_transformation) and the transformation maps the circle circumscribing the 3 points to a circle of infinite radius, which directly implies collinearity of the reciprocals.

However, this is assuming that the original circle circumscribing z_1, z_2 and z_3 is itself a circle, which is trivial.

cutemouse · Feb 9, 2012

Re: 2012 HSC MX2 Marathon

Carrotsticks said:
Proof #2

We recognise that the mapping...

$z \rightarrow \frac{1}{z}$

... is a Mobius Transform (http://en.wikipedia.org/wiki/Möbius_transformation) and the transformation maps the circle circumscribing the 3 points to a circle of infinite radius, which directly implies collinearity of the reciprocals.

However, this is assuming that the original circle circumscribing z_1, z_2 and z_3 is itself a circle, which is trivial.

That is what I was looking for, but at a more elementary level.

Carrotsticks · Feb 9, 2012

Re: 2012 HSC MX2 Marathon

I just realised that I said "assuming the original circle circumscribing z_1, z_2 and z_3 is itself a circle"

I meant "Generalised Circle".

HSC 2012 MX2 Marathon (archive) (1 Viewer)

Well-Known Member

Retired

Well-Known Member

Member

Well-Known Member

Retired

Member

Well-Known Member

Retired

Member

Premium Member

Member

Retired

Account Closed

Retired

Member

Retired

Retired

Account Closed

Retired

Users Who Are Viewing This Thread (Users: 0, Guests: 1)