HSC 2014 MX2 Marathon ADVANCED (archive) (6 Viewers)

mreditor16 · Oct 3, 2014

Re: HSC 2014 4U Marathon - Advanced Level

glittergal96 said:
To be honest, I actually forgot to register lol. For some reason I had it in my head that it was next week :/.

No biggie though, I will just do the exam at home later whenever it gets uploaded.

me too

Chlee1998 · Oct 5, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Given that a + b + c= 3

Prove that a^2 + b^2 + c^2 + ab+ bc+ca>=6

Sy123 · Oct 6, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Chlee1998 said:
Given that a + b + c= 3

Prove that a^2 + b^2 + c^2 + ab+ bc+ca>=6

(Assuming a,b,c are non-negative)

$\\ $Make the substitution$ \ x = a/3, y = b/3, z = c/3 \\ $So$ \ x+y+z = 1 \\ $And the problem is transformed into proving that$ \\ \\ xy+ xz + yz \leq \frac{1}{3} \ \ $(after using expansion of$ \ (x+y+z)^2 \ $)$ \\ \\ $Now$ \ xy + z(x+y) \leq \frac{1}{3} \Rightarrow \ xy + (x+y) - (x+y)^2 \leq \frac{1}{3} \ $(using the fact that$ \ z = 1 - (x+y) \ $)$ \\ \\ \Rightarrow \ \frac{1}{3} + (x^2+xy+y^2) \geq (x+y)$

$\\ $Without loss of generality let$ \ x > y \\ \\ $Then$ \ \frac{1}{3} + \frac{x^3-y^3}{x-y} \geq x+y \\ \\ \Rightarrow \ x^3 - x^2 + \frac{1}{3}x \geq y^3 - y^2 + \frac{1}{3}y \ \ $(*) (this remains to be proven)$$

$\\ $Take the polynomial$ \ P(x) = x^3 - x^2 + \frac{1}{3}x \\ \\ P'(x) = \left( \sqrt{3}x + \frac{1}{\sqrt{3}} \right)^2 > 0 \\ \\ $Thus$ \ P \ $is monotone increasing, meaning that if$ \ x > y \ $then$ \ P(x) > P(y) \ $therefore proving$ \ (*)$

$\\ $Take the case that$ \ x = y \\ \\ \Rightarrow \ 3x^2 - 2x + \frac{1}{3} \geq 0 \Rightarrow \ \left(\sqrt{3}x - \frac{1}{\sqrt{3}} \right)^2 \geq 0 \ $which is true$$

$\\ $Thus for all cases, the inequality$ \ xy + xz + zx \leq \frac{1}{3} \ $proving the original inequality$$

Sy123 · Oct 8, 2014

Re: HSC 2014 4U Marathon - Advanced Level

$\\ $Given$ \ a+b+c = 1 \ \\ \\ $Prove that$ \ a\sqrt[3]{1+b-c} + b\sqrt[3]{1+c-a} + c\sqrt[3]{1+a-b} \leq 1$

glittergal96 · Oct 9, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Sy123 said:
$\\ $Given$ \ a+b+c = 1 \ \\ \\ $Prove that$ \ a\sqrt[3]{1+b-c} + b\sqrt[3]{1+c-a} + c\sqrt[3]{1+a-b} \leq 1$

Consider $f(t)=t^3$ for $t>0$ . This function is concave up, and so every secant of its graph lies above the graph itself.

This means that for $x,y\geq 0$ and $s+t=1$ , we have $f(sx+ty)\leq sf(x)+tf(y)$ . (The sum 1 condition means that we are doing an internal division of a line segment.)

Now for a given $x,y,z \geq 0$ and $a+b+c=1$ , we have

$f(ax+by+cz)=f(ax+(b+c)(\frac{b}{b+c}y+\frac{c}{b+c}z))$

$\leq af(x)+ (b+c)f(\frac{b}{b+c}y+\frac{c}{b+c}z)\leq af(x)+bf(y)+cf(z).$

In other words we have

$(ax+by+cz)\leq(ax^3+by^3+cz^3)^{1/3}.$

Applying this to the LHS of the inequality we are trying to prove and we get

$LHS \leq (a(1+b-c)+b(1+c-a)+c(1+a-b))^{1/3} =(a+b+c)^{1/3}=1=RHS.$

Sy123 · Oct 9, 2014

Re: HSC 2014 4U Marathon - Advanced Level

glittergal96 said:
Consider $f(t)=t^3$ for $t>0$ . This function is concave up, and so every secant of its graph lies above the graph itself.

This means that for $x,y\geq 0$ and $s+t=1$ , we have $f(sx+ty)\leq sf(x)+tf(y)$ . (The sum 1 condition means that we are doing an internal division of a line segment.)

Now for a given $x,y,z \geq 0$ and $a+b+c=1$ , we have

$f(ax+by+cz)=f(ax+(b+c)(\frac{b}{b+c}y+\frac{c}{b+c}z))$

$\leq af(x)+ (b+c)f(\frac{b}{b+c}y+\frac{c}{b+c}z)\leq af(x)+bf(y)+cf(z).$

In other words we have

$(ax+by+cz)\leq(ax^3+by^3+cz^3)^{1/3}.$

Applying this to the LHS of the inequality we are trying to prove and we get

$LHS \leq (a(1+b-c)+b(1+c-a)+c(1+a-b))^{1/3} =(a+b+c)^{1/3}=1=RHS.$

Nice
My alternate solution:

$\\ LHS = \sqrt[3]{a^3(a+2b)} + \sqrt[3]{b^3(b+2c)} + \sqrt[3]{c^3(c+2a)} \leq \frac{1}{3}(a(a+2b) + 2a) + \frac{1}{3}(b(b+2c) + 2b) + \frac{1}{3}(c(c+2a) + 2c) \\ \\ = \frac{1}{3}(2(a+b+c) + (a+b+c)^2) = 1 \ \square$

Chlee1998 · Oct 9, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Sy123 said:
(Assuming a,b,c are non-negative)

$\\ $Make the substitution$ \ x = a/3, y = b/3, z = c/3 \\ $So$ \ x+y+z = 1 \\ $And the problem is transformed into proving that$ \\ \\ xy+ xz + yz \leq \frac{1}{3} \ \ $(after using expansion of$ \ (x+y+z)^2 \ $)$ \\ \\ $Now$ \ xy + z(x+y) \leq \frac{1}{3} \Rightarrow \ xy + (x+y) - (x+y)^2 \leq \frac{1}{3} \ $(using the fact that$ \ z = 1 - (x+y) \ $)$ \\ \\ \Rightarrow \ \frac{1}{3} + (x^2+xy+y^2) \geq (x+y)$

$\\ $Without loss of generality let$ \ x > y \\ \\ $Then$ \ \frac{1}{3} + \frac{x^3-y^3}{x-y} \geq x+y \\ \\ \Rightarrow \ x^3 - x^2 + \frac{1}{3}x \geq y^3 - y^2 + \frac{1}{3}y \ \ $(*) (this remains to be proven)$$

$\\ $Take the polynomial$ \ P(x) = x^3 - x^2 + \frac{1}{3}x \\ \\ P'(x) = \left( \sqrt{3}x + \frac{1}{\sqrt{3}} \right)^2 > 0 \\ \\ $Thus$ \ P \ $is monotone increasing, meaning that if$ \ x > y \ $then$ \ P(x) > P(y) \ $therefore proving$ \ (*)$

$\\ $Take the case that$ \ x = y \\ \\ \Rightarrow \ 3x^2 - 2x + \frac{1}{3} \geq 0 \Rightarrow \ \left(\sqrt{3}x - \frac{1}{\sqrt{3}} \right)^2 \geq 0 \ $which is true$$

$\\ $Thus for all cases, the inequality$ \ xy + xz + zx \leq \frac{1}{3} \ $proving the original inequality$$

Just an alternative,
from (a+b+c)(1/a + 1/b+ 1/c) >=9, 1/a + 1/b + 1/c >=3

From AM GM, a+b+c/3 = 1> (abc)^1/3. Thus abc < 1

transform LHS into (a+b+c)^2 - ab -bc -ca.

which equals 9 - abc(1/a + 1/b + 1/c) >= 9-3 =6

RealiseNothing · Oct 10, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Chlee1998 said:
from (a+b+c)(1/a + 1/b+ 1/c) >=9, 1/a + 1/b + 1/c >=3

From AM GM, a+b+c/3 = 1> (abc)^1/3. Thus abc < 1

transform LHS into (a+b+c)^2 - ab -bc -ca.

which equals 9 - abc(1/a + 1/b + 1/c) >= 9-3 =6

You barely proved half the things you claimed.

Chlee1998 · Oct 11, 2014

Re: HSC 2014 4U Marathon - Advanced Level

RealiseNothing said:
You barely proved half the things you claimed.

A fair criticism. However if you look at Sys previous post he didnt include a proof for am gm. So I assumed on this forum I was could assume the really common theorems. Of course in an exam i wouldve most definity included proofs. But i didnt think including them here would be necessary.

RealiseNothing · Oct 11, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Chlee1998 said:
A fair criticism. However if you look at Sys previous post he didnt include a proof for am gm. So I assumed on this forum I was could assume the really common theorems. Of course in an exam i wouldve most definity included proofs. But i didnt think including them here would be necessary.

I wasn't referring to AM-GM.

$abc(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}) \leq 3$ for example

Also $(a+b+c)(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}) \geq 9$

Chlee1998 · Oct 11, 2014

Re: HSC 2014 4U Marathon - Advanced Level

RealiseNothing said:
I wasn't referring to AM-GM.

$abc(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}) \leq 3$ for example

Also $(a+b+c)(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}) \geq 9$

whoops i just realised the previous method didn't quite work. Thanks Realise.

But I just thought of another method.

Change the LHS into (3-c)^2 + (3-a) ^2 + (3-b)^2
The goal is to prove that this is greater than 12
After expanding and cancelling off terms, you are left with proving that a^2 + b^2 +c^2 > a+ b+ c
Which comes really simply from a^2 + 1 > 2a (AM GM)
and b^2 + 1 > 2b
c^2 + 1 > 2c

Suming all three of these gives a^2 + b^2 + c^2 + 3 > 2a + 2b + 2c
and considering that a+b+c =3,
a^2 + b^2 +c^2 > a+ b+c.

Hence proven.
I am open to your criticism Realise

abecina · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

not sure which forum to post this. Hope this is ok

glittergal96 · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

abecina said:
not sure which forum to post this. Hope this is ok

The number of orbits at such a time t must differ by integral amounts, so we have

t/p = t/p^2 + k

and

t/p^2 = t/p^3 + l

for some positive integers k,l. (Positive because their different periods means they definitely can't have had the same number of orbits at any positive time.)

Solving these for t, we get

t= kp^2/(p-1) = lp^3/(p-1).

As l must be at least 1, t must be at least p^3/(p-1). This is in fact possible, because we can take k=p to ensure that the middle quantity is also equal.

So the time sought is p^3/(p-1).

abecina · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

part ii)?

glittergal96 · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

abecina said:
part ii)?

Oh didn't see that! Does it just mean that the 3 are in a line, with some on opposite sides of the star? Will do this in a bit, just about to grab dinner

.

abecina · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

yep that's right

glittergal96 · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

abecina said:
yep that's right

Ah okay, then the same thing works really, you just have to replace the "differ by integers" with "differ by half-integers".

We get $t=\frac{p^2k}{2(p-1)}=\frac{p^3l}{2(p-1)}$

which has minimal solution

$t=\frac{p^3}{2(p-1)}.$

abecina · Oct 15, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Good job on pt i) and ii): for pt ii) I did if $\textrm {Let } T^* \textrm{ be first time for planets to be aligned, one on either endpoint. Then } 2T^* \textrm{ will be the first time planets are aligned with planets on same side.} \therefore 2T^* = \frac{p^3}{p-1} \implies T^* = \frac{p^3}{2(p-1)}$

abecina · Oct 16, 2014

Re: HSC 2014 4U Marathon - Advanced Level

Here's a follow up question.

A clock has an hour-hand of 3 units and a minute-hand of 4 units. If the hands are currently positioned at three-o'-clock, determine the first time when the tips of the hands are moving away from each other the fastest

glittergal96 · Oct 18, 2014

Re: HSC 2014 4U Marathon - Advanced Level

abecina said:
Here's a follow up question.

A clock has an hour-hand of 3 units and a minute-hand of 4 units. If the hands are currently positioned at three-o'-clock, determine the first time when the tips of the hands are moving away from each other the fastest

This is a bit tedious, so I won't include every detail, but I think this is right.

We can write the two hands endpoints in polar coordinates as

$(r_1,\theta_1)=(3,\pi/2+2\pi t) \quad (r_2,\theta_2)=(4,120\pi t).$

As the hands have constant angular speeds, and hand 2 is moving faster, the first time the tips are moving away the fastest will occur when

$\theta_2-\theta_1\in [0,\pi] \Rightarrow 118t \in [1/2,3/2]\quad (*).$

We write $\phi=118\pi t$ for brevity. (This is the angle between hands, offset by a constant factor of $\pi/2$ .)

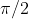
If x is the separating distance, we have (using the cosine rule),

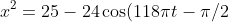$x^2=25-24\cos(118\pi t - \pi/2)=25-24\sin(\phi).$

Differentiating twice using the chain rule we get

$2xx'=-24\cdot 118\pi\cdot \cos(\phi)$

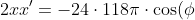and

$2(x')^2+2xx''=24\cdot(118\pi)^2\cdot \sin(\phi).$

Now, at the time of interest, x'' vanishes, so plugging the expression for the first derivative of x into the second equation and simplifying we get

$12\cos^2(\phi)=25\sin(\phi)-24\sin^2(\phi).$

Use the Pythagorean identity to put everything in terms of sin(x), and factorise the resulting quadratic to get.

$(3\sin(\phi)-4)(4\sin(\phi)-3)=0\Rightarrow \sin(\phi)=3/4.$

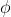
Since $\phi$ must lie in one of the second two quadrants (*), this gives

$t=\frac{\pi-\arcsin(3/4)}{118\pi}.$

(Here t is the number of hours elapsed since the starting time of 3:00.)

HSC 2014 MX2 Marathon ADVANCED (archive) (6 Viewers)

Well-Known Member

Member

This too shall pass

This too shall pass

Active Member

This too shall pass

Member

what is that?It is Cowpea

Member

what is that?It is Cowpea

Member

Member

Attachments

Active Member

Member

Active Member

Member

Active Member

Member

Member

Active Member

Users Who Are Viewing This Thread (Users: 0, Guests: 6)