Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
HSC 2015 MX2 Marathon (archive) (7 Viewers)
- Thread starter Trebla
- Start date
- Status
- Not open for further replies.
porcupinetree
not actually a porcupine
- Joined
- Dec 12, 2014
- Messages
- 661
- Gender
- Male
- HSC
- 2015
Ekman
Well-Known Member
- Joined
- Oct 23, 2014
- Messages
- 1,611
- Gender
- Male
- HSC
- 2015
Last edited:
porcupinetree
not actually a porcupine
- Joined
- Dec 12, 2014
- Messages
- 661
- Gender
- Male
- HSC
- 2015
Ekman
Well-Known Member
- Joined
- Oct 23, 2014
- Messages
- 1,611
- Gender
- Male
- HSC
- 2015
Re: HSC 2015 4U Marathon

Then I used limiting sum to get:
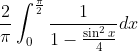
I converted the sum into integral format:How did you do this?
Then I used limiting sum to get:
porcupinetree
not actually a porcupine
- Joined
- Dec 12, 2014
- Messages
- 661
- Gender
- Male
- HSC
- 2015
Re: HSC 2015 4U Marathon
And then how do you evaluate that integral? t method seems a little tedious, is there a simpler way?I converted the sum into integral format:
Then I used limiting sum to get:
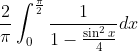
Sy123
This too shall pass
- Joined
- Nov 6, 2011
- Messages
- 3,725
- Gender
- Male
- HSC
- 2013
Sy123
This too shall pass
- Joined
- Nov 6, 2011
- Messages
- 3,725
- Gender
- Male
- HSC
- 2013
Re: HSC 2015 4U Marathon
A translation of part (ii) is simply "show that no two members of the sequence F_n share a common factor"
bump
A translation of part (ii) is simply "show that no two members of the sequence F_n share a common factor"
Re: HSC 2015 4U Marathon
Isn't this essentially a derivation of the cubic formula? That is famously messy lol.
Drsoccerball
Well-Known Member
- Joined
- May 28, 2014
- Messages
- 3,647
- Gender
- Undisclosed
- HSC
- 2015
Re: HSC 2015 4U Marathon
Theyre all prime numbersbump
A translation of part (ii) is simply "show that no two members of the sequence F_n share a common factor"
Re: HSC 2015 4U Marathon
E.g.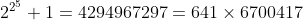 .
.
Not all of them are; the first several are, but the pattern breaks down after a point.Theyre all prime numbers
E.g.
Last edited:
Ekman
Well-Known Member
- Joined
- Oct 23, 2014
- Messages
- 1,611
- Gender
- Male
- HSC
- 2015
Re: HSC 2015 4U Marathon


 $ \therefore \frac{\ F_0F_1\dots F_{I} \dots F_{j-1}}{F_j} $ is not an integer $ )
Edit: Nevermind, I only proved that they are not divisible, I didn't prove that they didn't have a common factor.
For part ii:
Edit: Nevermind, I only proved that they are not divisible, I didn't prove that they didn't have a common factor.
Last edited:
RealiseNothing
what is that?It is Cowpea
RealiseNothing
what is that?It is Cowpea
Re: HSC 2015 4U Marathon
You're pretty close though.Edit: Nevermind, I only proved that they are not divisible, I didn't prove that they didn't have a common factor.
Sy123
This too shall pass
- Joined
- Nov 6, 2011
- Messages
- 3,725
- Gender
- Male
- HSC
- 2013
Re: HSC 2015 4U Marathon
Try proving via contradictionFor part ii:
Edit: Nevermind, I only proved that they are not divisible, I didn't prove that they didn't have a common factor.
Ekman
Well-Known Member
- Joined
- Oct 23, 2014
- Messages
- 1,611
- Gender
- Male
- HSC
- 2015
Re: HSC 2015 4U Marathon
You're pretty close though.
I'm assuming you would use a similar method but instead of using the numbers F_n, you would use the factors of those integers, and prove that the factors cannot be integers, hence proving they do not share common factors.Try proving via contradiction
- Status
- Not open for further replies.
