HSC 2013 MX2 Marathon (archive) (1 Viewer)

shongaponga · May 11, 2013

Re: HSC 2013 4U Marathon

bleakarcher said:
Did it. ^ That happened.

You copied the wrong code, swing me a PM so we aren't spamming this thread and i'll teach you how to do it.

Registered User · May 11, 2013

Re: HSC 2013 4U Marathon

$Prove the inequality:$

${a^{2}}+b^2+c^2+\frac{2}{5}abc<50$

$where:$

$a,b,c are the lengths of triangle's sides$

$and the perimeter of the triangle is 10.$

Registered User · May 12, 2013

Re: HSC 2013 4U Marathon

$Prove that$

$\prod_{k=1}^{n}\left ( 1+2cos\frac{2\pi .3^{k}}{3^{n}+1} \right )=1$

Registered User · May 12, 2013

Re: HSC 2013 4U Marathon

$Prove that$

$\left ( \frac{1}{2}+cos\frac{\pi }{20} \right )\left ( \frac{1}{2}+cos\frac{3\pi }{20} \right )\left ( \frac{1}{2}+cos\frac{9\pi }{20} \right )\left ( \frac{1}{2}+cos\frac{27\pi }{20} \right )=\frac{1}{16}$

Registered User · May 12, 2013

Re: HSC 2013 4U Marathon

bleakarcher said:
1) [(1/a)-(1/b)]^2 + [(1/a)-(1/c)]^2 + [(1/b)-(1/c)]^2 >=0 (equality occurs iff a=b=c)
2/a^2 + 2/b^2 + 2/c^2 -2[(1/ab)+(1/bc)+(1/ac)] >=0
=> (1/a)^2 + (1/b)^2 + (1/c)^2 >= 1/bc + 1/ac + 1/ab for all real a,b,c=/=0 (*)
Hence, bc/a + ac/b + ab/c = abc[(1/a^2)+(1/b^2) +(1/c^2)]>=abc[(1/ab)+(1/bc)+(1/ac)]=a+b+c as req.
2) Refer back to (*), replace a with sqrt(a), b with sqrt(b) and c with sqrt(c) and the second inequality immediately falls out for a,b,c>0

Sorry if it's hard to read, still haven't learnt latex.

$1)\ (\frac{1}{a}-\frac{1}{b})^{2} + (\frac{1}{a}-\frac{1}{c})^{2} + (\frac{1}{b}-\frac{1}{c})^{2} > 0$ $(equality occurs iff a=b=c)$

$\frac{2}{a^{2}} + \frac{2}{b^{2}} + \frac{2}{c^{2}} -2\left ( \frac{1}{ab}+\frac{1}{bc}+\frac{1}{ac} \right ) \geq 0$

$\Rightarrow \left ( \frac{1}{a} \right )^{2} + \left ( \frac{1}{b} \right )^{2} + \left ( \frac{1}{c} \right )^{2} \geq \frac{1}{bc} + \frac{1}{ac} + \frac{1}{ab}$ $for all real$ $a,b,c\neq 0 (*)$

$Hence,$ $\frac{bc}{a} + \frac{ac}{b} + \frac{ab}{c} = abc\left ( \frac{1}{a^{2}}+ \frac{1}{b^{2}} +\frac{1}{c^{2}} \right )\geq \frac{bc}{a}+\frac{ac}{b}+\frac{ab}{c}\geqslant (a+b+c)$ $as required.$

$2) Refer back to (*), replace a with$ $\sqrt{a},$ $b with$ $\sqrt{b},$ $and c with$ $\sqrt{c}$ $and the second inequality immediately falls out for$ $a,b,c > 0$

http://www.codecogs.com/latex/eqneditor.php

Make the latex there then put $[/ tex](*) For writing put [tex]$ writing $[/ tex] (*) Without the space$

Registered User · May 12, 2013

Re: HSC 2013 4U Marathon

$For any positive real numbers$ $a,b,c$ $show that the following inequality holds$ $\left ( \frac{a}{b}+\frac{b}{c}+\frac{c}{a} \right )\geq \left ( \frac{c+a}{c+b}+\frac{a+b}{a+c}+\frac{b+c}{b+a} \right )$

Sy123 · May 12, 2013

Re: HSC 2013 4U Marathon

Registered User said:
$Prove that$

$\prod_{k=1}^{n}\left ( 1+2cos\frac{2\pi .3^{k}}{3^{n}+1} \right )=1$

$\sin(3\theta) = \sin \theta \cos(2\theta) + \cos \theta \sin(2\theta)$

$\frac{\sin 3\theta}{\sin \theta} = \cos 2\theta + 2\cos^2 \theta$

$\therefore \ \ \frac{\sin 3\theta}{\sin \theta} = 1+ 2\cos 2\theta \ \ \dots \ \ (1)$

$$Let$ \ \ \theta_k = \frac{\pi 3^k}{3^n+1}$

$$Due to$ \ \ (1)$

$\therefore \ \ P = \prod_{k=1}^{n} \left (1+ 2\cos 2\theta_k \right ) = \frac{\sin 3\theta_1}{\sin \theta_1} \cdot \frac{\sin 3\theta_2}{\sin \theta_2} \dots \frac{\sin 3\theta_n}{\sin \theta_n}$

$\theta_k = 3\theta_{k-1}$

$A 'telescoping product' is formed$

$P = \frac{\sin \theta_{n+1}}{\sin \theta_1}$

$\therefore P = \prod_{k=1}^{n} \left (1+ 2\cos 2\theta_k \right ) = \frac{\sin \left (3\pi - \theta_1 \right)}{\sin (\theta_1)} = 1$

$\square$

Registered User · May 13, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$\sin(3\theta) = \sin \theta \cos(2\theta) + \cos \theta \sin(2\theta)$

$\frac{\sin 3\theta}{\sin \theta} = \cos 2\theta + 2\cos^2 \theta$

$\therefore \ \ \frac{\sin 3\theta}{\sin \theta} = 1+ 2\cos 2\theta \ \ \dots \ \ (1)$

$$Let$ \ \ \theta_k = \frac{\pi 3^k}{3^n+1}$

$$Due to$ \ \ (1)$

$\therefore \ \ P = \prod_{k=1}^{n} \left (1+ 2\cos 2\theta_k \right ) = \frac{\sin 3\theta_1}{\sin \theta_1} \cdot \frac{\sin 3\theta_2}{\sin \theta_2} \dots \frac{\sin 3\theta_n}{\sin \theta_n}$

$\theta_k = 3\theta_{k-1}$

$A 'telescoping product' is formed$

$P = \frac{\sin \theta_{n+1}}{\sin \theta_1}$

$\therefore P = \prod_{k=1}^{n} \left (1+ 2\cos 2\theta_k \right ) = \frac{\sin \left (3\pi - \theta_1 \right)}{\sin (\theta_1)} = 1$

$\square$

Nice work.

Registered User · May 14, 2013

Re: HSC 2013 4U Marathon

$Prove that$

$\frac{ \sin x}{ \cos x}+\frac{\sin2x}{\cos^{2}x}+\frac{\sin3x}{\cos^{3}x}+\cdots+\frac{\sin nx}{\cos^{n}x}=\cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

Do not use induction.

Sy123 · May 14, 2013

Re: HSC 2013 4U Marathon

Registered User said:
$Prove that$

$\frac{ \sin x}{ \cos x}+\frac{\sin2x}{\cos^{2}x}+\frac{\sin3x}{\cos^{3}x}+\cdots+\frac{\sin nx}{\cos^{n}x}=\cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

Do not use induction.

$By DeMoivere's theorem$

$(\cos x + i\sin x)^k = \cos(kx) + i\sin(kx)$

$(1+ i\tan x)^k = \frac{\cos(kx)}{\cos^kx} + i \frac{\sin(kx)}{\cos^k x}$

$$Im$ \left(\sum_{k=1}^{n} (1+i\tan x)^k \right) = \sum_{k=1}^{n} \frac{\sin kx}{\cos^kx} \ \ \dots \ \ (1)$

$Consider the geometric series$

$(1+i\tan x) + (1+i\tan x)^2 + \dots + (1+i\tan x)^n = \frac{(1+i\tan x)^{n+1} - (1+i\tan x)}{1+i\tan x - 1}$

$\Rightarrow \ \ $Im$ \frac{\frac{\cos(n+1)x}{\cos^{n+1}x} + \frac{i\sin(n+1)x}{\cos^{n+1}x} - 1 - i\tan x}{i\tan x} = \cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

$\therefore \ \frac{ \sin x}{ \cos x}+\frac{\sin2x}{\cos^{2}x}+\frac{\sin3x}{\cos^{3}x}+\cdots+\frac{\sin nx}{\cos^{n}x}=\cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

bleakarcher · May 14, 2013

Re: HSC 2013 4U Marathon

Hey guys, here's a question from last year's UNSW maths competition. Let f(x) denote a strictly positive continuous function defined on all real numbers with the property that f(2012)=2012 and f(x)=f(x+f(x)) for all x. Prove that f(x)=2012 for all x.

Sy123 · May 14, 2013

Re: HSC 2013 4U Marathon

bleakarcher said:
Hey guys, here's a question from last year's UNSW maths competition. Let f(x) denote a strictly positive continuous function defined on all real numbers with the property that f(2012)=2012 and f(x)=f(x+f(x)) for all x. Prove that f(x)=2012 for all x.

$$For some number$ \ \ a$

$f(a) = f(a+f(a+f(a+f(a+ \dots +f(a)) \dots ) = f(k)$ for some number k, f is continuous and defined on all real therefore a and k can be anything.
If that is the case then a and k can be such that they represent any pair of real numbers. The function of these numbers are all equal, therefore

$f(x) = C$ for some constant C since f(a) = f(k)

$f(2012) = 2012$

$\therefore f(x) = 2012$

Is that good enough?

============

$Determine all solutions to the simultaneous equations$

$x+y+z = 3$

$x^2+y^2+z^2 = 3$

$x^3+y^3+z^3 = 3$

RealiseNothing · May 14, 2013

Re: HSC 2013 4U Marathon

edit: I get it.

Makematics · May 14, 2013

Re: HSC 2013 4U Marathon

bleakarcher said:
Hey guys, here's a question from last year's UNSW maths competition. Let f(x) denote a strictly positive continuous function defined on all real numbers with the property that f(2012)=2012 and f(x)=f(x+f(x)) for all x. Prove that f(x)=2012 for all x.

omg thank you so much for asking that. really wanted to know the solution

Registered User · May 14, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$By DeMoivere's theorem$

$(\cos x + i\sin x)^k = \cos(kx) + i\sin(kx)$

$(1+ i\tan x)^k = \frac{\cos(kx)}{\cos^kx} + i \frac{\sin(kx)}{\cos^k x}$

$$Im$ \left(\sum_{k=1}^{n} (1+i\tan x)^k \right) = \sum_{k=1}^{n} \frac{\sin kx}{\cos^kx} \ \ \dots \ \ (1)$

$Consider the geometric series$

$(1+i\tan x) + (1+i\tan x)^2 + \dots + (1+i\tan x)^n = \frac{(1+i\tan x)^{n+1} - (1+i\tan x)}{1+i\tan x - 1}$

$\Rightarrow \ \ $Im$ \frac{\frac{\cos(n+1)x}{\cos^{n+1}x} + \frac{i\sin(n+1)x}{\cos^{n+1}x} - 1 - i\tan x}{i\tan x} = \cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

$\therefore \ \frac{ \sin x}{ \cos x}+\frac{\sin2x}{\cos^{2}x}+\frac{\sin3x}{\cos^{3}x}+\cdots+\frac{\sin nx}{\cos^{n}x}=\cot x-\frac{\cos(n+1)x}{\sin x \cos^{n}x}$

Can also be done by expanding $cos(nx+x)$ but your method is nice too.

==============================

$What does$ $\sum_{k=0}^{n}k\times k!$ $converge to?$

RealiseNothing · May 14, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$Determine all solutions to the simultaneous equations$

$x+y+z = 3$

$x^2+y^2+z^2 = 3$

$x^3+y^3+z^3 = 3$

Is there any other solutions besides $x=y=z=1$ because my method yields that as the only solution.

Sy123 · May 14, 2013

Re: HSC 2013 4U Marathon

RealiseNothing said:
Is there any other solutions besides $x=y=z=1$ because my method yields that as the only solution.

Yep those are the only solutions, what was your method?

RealiseNothing · May 14, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
Yep those are the only solutions, what was your method?

My aim was to construct a cubic polynomial which has roots x, y, z, using sum of roots/product of roots/two at a time. Then by finding the roots of this polynomial, we find the values of x, y, z, that satisfy the system of equations. The cubic is in the form:

$X^3 - aX^2 + bX - c$

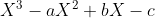
The sum of roots is easy as it's already given:

$x+y+z=~$sum of roots$~ = 3 = a$

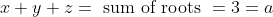
So we have:

$X^3 - 3X^2 + bX - c$

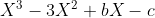
Now we get the value of 'b' by finding the sum of the pair roots (xy+xz+yz). We know that:

$x^2+y^2+z^2=(x+y+z)^2 - 2(xy+xz+yz)$

$3=3^2-2(xy+xz+yz)$

$xy+xz+yz = 3 = b$

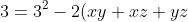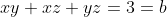
So we now have:

$X^3 - 3X^2 + 3X - c$

Now we know that x, y, z and the roots of this equation, so by substitution we arrive at:

$x^3 - 3x^2 + 3x - c = 0$

$y^3 - 3y^2 + 3y - c = 0$

$z^3 - 3z^2 + 3z - c = 0$

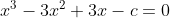
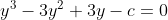
If we add all these together we get:

$(x^3+y^3+z^3) - 3(x^2+y^2+z^2) + 3(x+y+z) = 3c$

$(3)-3(3)+3(3) = 3c$

$c=1$

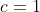
So our polynomial is:

$X^3-3X^2+3X-1$

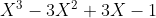
Which simplifies to:

$(X-1)^3 = 0$

Which has all roots equal to 1. So x, y, z are all equal to 1. And the polynomial can not have other roots and so this is the only possible solution set to the given system of equations.

bleakarcher · May 14, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$$For some number$ \ \ a$

$f(a) = f(a+f(a+f(a+f(a+ \dots +f(a)) \dots ) = f(k)$ for some number k, f is continuous and defined on all real therefore a and k can be anything.
If that is the case then a and k can be such that they represent any pair of real numbers. The function of these numbers are all equal, therefore

$f(x) = C$ for some constant C since f(a) = f(k)

$f(2012) = 2012$

$\therefore f(x) = 2012$

Is that good enough?

============

$Determine all solutions to the simultaneous equations$

$x+y+z = 3$

$x^2+y^2+z^2 = 3$

$x^3+y^3+z^3 = 3$

Think it works dude, nice. I'm not so sure about my method though:

f(x)=f(x+f(x))
f '(x)=[1+f '(x)]*f '(x+f(x))=[1+f '(x)]*f '(x) => f '(x)=0 for all x. Since f(x) is continuous and f(2012)=2012, f(x)=2012 for all x.

RealiseNothing · May 14, 2013

Re: HSC 2013 4U Marathon

bleakarcher said:
Think it works dude, nice. I'm not so sure about my method though:

f(x)=f(x+f(x))
f '(x)=[1+f '(x)]*f '(x+f(x))=[1+f '(x)]*f '(x) => f '(x)=0 for all x. Since f(x) is continuous, f(x)=2012 for all x.

How did you get that $f'(x+f(x)) = f'(x)$ ?

HSC 2013 MX2 Marathon (archive) (1 Viewer)

Member

Member

Member

Member

Member

Member

This too shall pass

Member

Member

This too shall pass

Active Member

This too shall pass

what is that?It is Cowpea

Well-Known Member

Member

what is that?It is Cowpea

This too shall pass

what is that?It is Cowpea

Active Member

what is that?It is Cowpea

Users Who Are Viewing This Thread (Users: 0, Guests: 1)