Similar to the Mathematics Thread started by ForrestGump at here.
How To Play:
The first person asks a Mathmatics Ext 1 question (maybe one you've had trouble with). The next person answers it then posts another question for the next person then so on.
Tip:
If you feel an area is your weakness then try asking a question on this area to see others responses to it which may help you in your understanding.
1st Question: (2 Marks)
Let=Ax^{3}-Ax+1) where
where 
Show that f(x) has exactly one zero when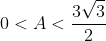
How To Play:
The first person asks a Mathmatics Ext 1 question (maybe one you've had trouble with). The next person answers it then posts another question for the next person then so on.
Tip:
If you feel an area is your weakness then try asking a question on this area to see others responses to it which may help you in your understanding.
1st Question: (2 Marks)
Let
Show that f(x) has exactly one zero when
Last edited:





